Lumos Video Store
This page provides a list of educational videos related to Shades of Word Meanings. You can also use this page to find sample questions, apps, worksheets, lessons , infographics and presentations related to Shades of Word Meanings.
Difference of Two Cubes | MathHelp.com

By MathHelp.com
To solve the given system of inequalities, we start by graphing the associated equation for each inequality. In other words, we graph y equals -1/5 x +1 and y equals 3x + 2. So, for the first inequality, we start with our y-intercept of positive 1, up 1 unit on the y-axis. From there, we take our slope of -1/5, so we go down 1 and to the right 5, and plot a second point. Now, notice that our inequality uses a “less than” sign. This means that we draw a dotted line connecting the points, rather than a solid line. It’s important to understand that if we have a greater than sign or a less than sign, we use a dotted line, and if we have a greater than or equal to sign or a less than or equal to sign, we use a solid line. Pay close attention to this idea when drawing your lines. Students often carelessly use a solid line when they should use a dotted one, and vice-versa. Next, let’s take a look at our second inequality, which has a y-intercept of positive 2, up 2 units on the y-axis. From there, we take our slope of 3, or 3 over 1, so we go up 3 and to the right 1, and plot a second point. And notice that this inequality uses a “greater than or equal to” sign, so we connect the points with a solid line, rather than a dotted line. Next, we need to determine which side of each of these lines to shade on the graph. To determine which side of our first line to shade, we use a test point on either side of the first line. The easiest test point to use is (0, 0), so we plug a zero into our first inequality for both x and y, and we have 0 is less than -1/5 times 0 + 1, which simplifies to 0 is less than 0 + 1, or 0 is less than 1. Notice that 0 is less than 1 is a true statement. This means that our test point, (0, 0), is a solution to the first inequality, so we shade in the direction of (0, 0) along our first boundary line. Next, we determine which side of our second line to shade by using a test point on either side of the second line, such as (0, 0). Plugging a zero into our second inequality for both x and y, we have 0 equal to 3 times 0 + 2, which simplifies to 0 equal to 0 + 2, or 0 equal to 2. Notice that 0 equal to 2 is a false statement. This means that our test point, (0, 0), is a not solution to the inequality, so we shade away from (0, 0) along our second boundary line. Finally, it’s important to understand that the solution to this system of inequalities is represented by the part of the graph where the two shaded regions overlap, which in this case is in the lower left. In other words, any point that lies in this part of the graph is a solution to the given system of inequalities. Note that the points along the dotted boundary line of this region are not solutions to the system, but the points along the solid boundary line of this region are solutions to the system.
Work Word Problems | MathHelp.com

By MathHelp.com
To solve a polynomial inequality, like the one shown here, our first step is to write the corresponding equation. In other words, we simply change the inequality sign to an equals sign, and we have x^2 – 3 = 9 – x. Next, we solve the equation. Since we have a squared term, we first set the equation equal to 0. So we move the 9 – x to the left side by subtracting 9 and adding x to both sides of the equation. This gives us x^2 + x – 12 = 0. Next, we factor the left side as the product of two binomials. Since the factors of negative 12 that add to positive 1 are positive 4 and negative 3, we have x + 4 times x – 3 = 0. So either x + 4 = 0 or x – 3 = 0, and solving each equation from here, we have x = -4, and x = 3. Now, it’s important to understand that the solutions to the equation, -4 and 3, represent what are called the “critical values” of the inequality, and we plot these critical values on a number line. However, notice that our original inequality uses a greater than sign, rather than greater than or equal to sign, so we use open dots on our critical values of -4 and positive 3. Remember that ‘greater than’ or ‘less than’ means open dot, and ‘greater than or equal to’ or ‘less than or equal to’ means closed dot. Now, we can see that our critical values have divided the number line into three separate intervals: less than -4, between -4 and 3, and greater than 3. And here’s the important part. Our next step is to test a value from each of the intervals by plugging the value back into the original inequality to see if it gives us a true statement. So let’s first test a value from the “less than -4” interval, such as -5. If we plug a -5 back in for both x’s in the original inequality, we have -5 squared – 3 greater than 9 minus a -5, which simplifies to 25 – 3 greater than 9 + 5, or 22 greater than 14. Since 22 greater than 14 is a true statement, this means that all values in the interval we’re testing are solutions to inequality, so we shade the interval. Next, we test a value from the “between -4 and 3” interval, such as 0. If we plug a 0 back in for both x’s in the original inequality, we have 0 squared – 3 greater than 9 – 0, which simplifies to 0 – 3 greater than 9, or -3 greater than 9. Since -3 greater than 9 is a false statement, this means that all values in the interval we’re testing are not solutions to inequality, so we don’t shade the interval. Next, we test a value from the “greater than 3” interval, such as 4. If we plug a 4 back in for both x’s in the original inequality, we have 4 squared – 3 greater than 9 – 4, which simplifies to 16 – 3 greater than 5, or 13 greater than 5. Since 13 greater than 5 is a true statement, this means that all values in the interval we’re testing are solutions to inequality, so we shade the interval. Finally, we write the answer that’s shown on our graph in set notation. The set of all x’s such that x is less than -4 or x is greater than 3.
11 - Learn ArcSin, ArcCos & ArcTan (Inverse Sin, Cos & Tan) - Part 1

By Math and Science
Quality Math And Science Videos that feature step-by-step example problems!
Area Between Two Curves

By The Organic Chemistry Tutor
This calculus video tutorial provides a basic introduction in finding the area between two curves with respect to y and with respect to x. It explains how to set up the definite integral to calculate the area of the shaded region bounded by the two curves. In order to find the points of intersection, you need to set the two curves equal to each other and solve for x or y. You need to be familiar with some basic integration techniques for this lesson. This video contains plenty of examples and practice problems.
16 - What do Imaginary & Complex Roots of Equations Mean?
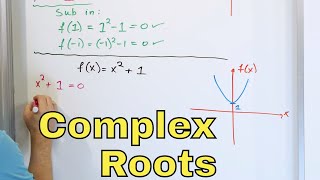
By Math and Science
Quality Math And Science Videos that feature step-by-step example problems!
Earth Science for Kids - Solar System, Weather, Fossils, Volcanoes & More - Rock 'N Learn

By Lumos Learning
Earth Science for Kids is the fun way to learn important facts about earth science and prepare for tests. Take a fascinating journey to the Super Science Station to learn about the Solar System, weather and the water cycle, types of rocks, properties of minerals, volcanoes and plate tectonics, and renewable energy. For gifted preschool learners to kids in junior high, all ages will enjoy this learning adventure. Clear explanations and humor make this video perfect for the classroom and home. Created along with teachers to meet state testing standards.
Micro and Alternative Credentials - Webinar 2

By Lumos Learning
Micro-credentials have emerged over the past five years in response to the increasing need for smaller, more frequent and more focused learning opportunities that attract academic or industry recognition. However, the micro-credential space is still in a formative state with no universally agreed format or definition, and with many participating actors and emerging models. This session provides an overview of HolonIQ's comprehensive analysis of the micro-credentials market globally, including definition and segmentation of the micro-credentials market, the emerging infrastructure to support micro-credential ecosystems, and analysis of the likely future role of micro-credentials in the post-secondary landscape.








