Lumos Video Store
This page provides a list of educational videos related to 9 as a Factor. You can also use this page to find sample questions, apps, worksheets, lessons , infographics and presentations related to 9 as a Factor.
Factor perfect squares

By Khan Academy
Sal finds the binomial factor shared by 4x^2+12x+9 and 4x^2-9.
Factoring algebraic expressions using the distributive property

By Khan Academy
Sal shows how to factor the expression 4x+18 into the expression 2(2x+9).
Factor polynomials with special product forms

By Khan Academy
Sal factors 25x^4-30x^2+9 as (5x^2-3)^2.
Factor polynomials with special product forms

By Khan Academy
Sal factors 25x^4-30x^2+9 as (5x^2-3)^2.
Factor polynomials with special product forms

By Khan Academy
Sal factors 25x^4-30x^2+9 as (5x^2-3)^2.
Factor polynomials with special product forms

By Khan Academy
Sal factors 25x^4-30x^2+9 as (5x^2-3)^2.
Factor polynomials with special product forms

By Khan Academy
Sal factors 25x^4-30x^2+9 as (5x^2-3)^2.
Learn Greatest Common Factor (GCF) & Least Common Multiple (LCM) - [7]

By Math and Science
Quality Math And Science Videos that feature step-by-step example problems!
Work Word Problems | MathHelp.com

By MathHelp.com
To solve a polynomial inequality, like the one shown here, our first step is to write the corresponding equation. In other words, we simply change the inequality sign to an equals sign, and we have x^2 – 3 = 9 – x. Next, we solve the equation. Since we have a squared term, we first set the equation equal to 0. So we move the 9 – x to the left side by subtracting 9 and adding x to both sides of the equation. This gives us x^2 + x – 12 = 0. Next, we factor the left side as the product of two binomials. Since the factors of negative 12 that add to positive 1 are positive 4 and negative 3, we have x + 4 times x – 3 = 0. So either x + 4 = 0 or x – 3 = 0, and solving each equation from here, we have x = -4, and x = 3. Now, it’s important to understand that the solutions to the equation, -4 and 3, represent what are called the “critical values” of the inequality, and we plot these critical values on a number line. However, notice that our original inequality uses a greater than sign, rather than greater than or equal to sign, so we use open dots on our critical values of -4 and positive 3. Remember that ‘greater than’ or ‘less than’ means open dot, and ‘greater than or equal to’ or ‘less than or equal to’ means closed dot. Now, we can see that our critical values have divided the number line into three separate intervals: less than -4, between -4 and 3, and greater than 3. And here’s the important part. Our next step is to test a value from each of the intervals by plugging the value back into the original inequality to see if it gives us a true statement. So let’s first test a value from the “less than -4” interval, such as -5. If we plug a -5 back in for both x’s in the original inequality, we have -5 squared – 3 greater than 9 minus a -5, which simplifies to 25 – 3 greater than 9 + 5, or 22 greater than 14. Since 22 greater than 14 is a true statement, this means that all values in the interval we’re testing are solutions to inequality, so we shade the interval. Next, we test a value from the “between -4 and 3” interval, such as 0. If we plug a 0 back in for both x’s in the original inequality, we have 0 squared – 3 greater than 9 – 0, which simplifies to 0 – 3 greater than 9, or -3 greater than 9. Since -3 greater than 9 is a false statement, this means that all values in the interval we’re testing are not solutions to inequality, so we don’t shade the interval. Next, we test a value from the “greater than 3” interval, such as 4. If we plug a 4 back in for both x’s in the original inequality, we have 4 squared – 3 greater than 9 – 4, which simplifies to 16 – 3 greater than 5, or 13 greater than 5. Since 13 greater than 5 is a true statement, this means that all values in the interval we’re testing are solutions to inequality, so we shade the interval. Finally, we write the answer that’s shown on our graph in set notation. The set of all x’s such that x is less than -4 or x is greater than 3.
Partial Fraction Decomposion

By The Organic Chemistry Tutor
This precalculus video tutorial provides a basic introduction into partial fraction decomposition. The full version of this video contains plenty of examples and practice problems with repeated linear factors and repeated quadratic factors. Partial fraction decomposition is the process of taking a complex fraction and breaking it into multiple simpler fractions. It's the reverse of adding combining two fractions into a single fraction.
Converting Units With Conversion Factors - Metric System Review & Dimensional Analysis

By The Organic Chemistry Tutor
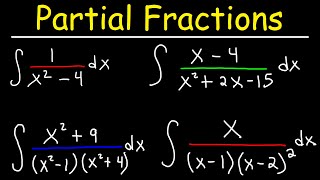
Integration By Partial Fractions
By The Organic Chemistry Tutor
This calculus video tutorial provides a basic introduction into integrating rational functions using the partial fraction decomposition method. Partial fraction decomposition is the process of breaking a single complex fraction into multiple simpler fractions. The integrals of many rational functions lead to a natural log function with absolute value expressions. This video explains what to do when you have repeated linear factors and quadratic factors. This tutorial contains many examples and practice problems on integration by partial fractions.